
Here, r is the common ratio between the consecutive terms.

To find the next term in a geometric sequence, we use the following formula The common difference can be calculated by subtracting any two consecutive terms. Here, t_1 is the first term of the sequence, n is the term number that we need to find, and d is the common difference between two consecutive terms. To find the next term in an arithmetic sequence, we use the following formula In geometric sequence or series, there is a constant ratio being followed between consecutive terms. The first difference is that the arithmetic sequence follows a constant difference between consecutive terms. So, what is the difference between these two basic types of sequences and series? The most basic ones are arithmetic and geometric. There are a variety of different types of these sequences and series. The series, on the other hand, is a process of adding infinitely many numbers without a fixed order.
SUM OF ARITHMETIC SEQUENCE WORKSHEET WITH ANSWERS PDF SERIES
When talking about sequence and series in mathematics, a sequence is a collection of numbers that are placed, following a specific order with repetitions allowed.
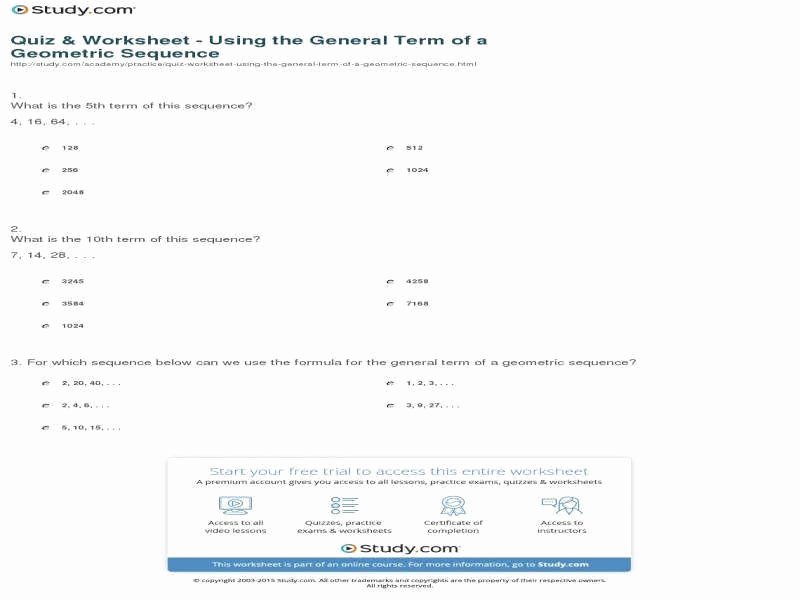
Series, on the other hand, is the arrangement of similar things one after the other, without following a fixed order. By sequence, we mean a list of things that obey a specific order. We come across the terms 'sequence' and 'series' very often in our lives.
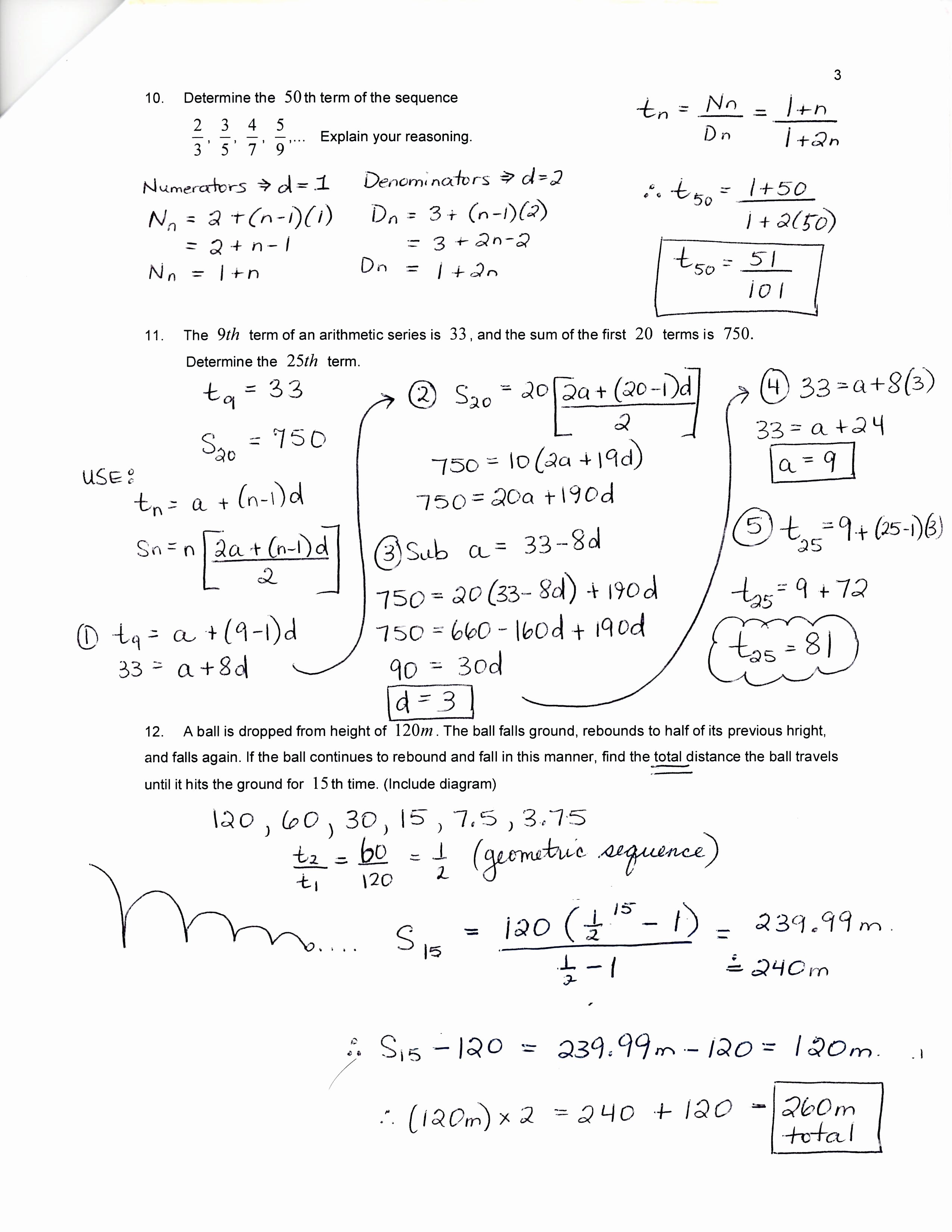
A geometric sequence is a sequence of numbers in which after the first term, consecutive ones are derived from multiplying the term before by a fixed, non-zero number called the common ratio. An arithmetic sequence is a sequence of numbers in which the interval between the consecutive terms is constant.


 0 kommentar(er)
0 kommentar(er)
